This short assignment on infinity is the last homework for the course. This assignment is due in class next Wednesday, December 10. Next week, there will be a few in-class group exercises related to polyhedra that will be turned in at the end of class.
We have been looking briefly in class at the idea of infinity. One way that mathematicians treat infinity is in terms of sets. A set is just a collection of things. In mathematics, sets of numbers and even sets of sets are possible. Thus, we have the set { 1, 2, 3 }, that consists of the numbers 1, 2, and 3. We have the set { 1, 2, 3, 4, ... }, which consists of all the positive integers. This latter set is an infinite set, since it contains more than any given finite number of items. (Of course when we say that this set "exists" as a real, complete thing, we are accepting the reality of an actual infinity, as opposed to just a potential infinity. Some philosophers, starting with Aristotle, were happy with the idea of potential infinity, but held that there is no such thing as an actual infinity. Modern mathematicians, for the most part, have come down strongly on the side of actual infinities.)
One thing that we can do with sets is to compare their sizes, that is, the number of items that they contain. It's natural to say that two sets have the same size if the items in the two sets can be matched up in pairs, with no items left over in either set. For example, the sets { 1, 2, 3, 4 } and { 5, 10, 15, 20 } have the same size because the items in the two sets can be matched up like this:
| 1 | 2 | 3 | 4 |
| | | | | | | | |
| 5 | 10 | 15 | 20 |
This idea can be extended to infinite sets. For example the infinite set of all positive integers, { 1, 2, 3, 4, ... }, and the infinite set of all even positive integers, { 2, 4, 6, 8, ... }, have the same size in this sense, since the items in the two sets can be paired up with no items left over in either set:
| 1 | 2 | 3 | 4 | 5 | 6 | . . . |
| | | | | | | | | | | | | |
| 2 | 4 | 6 | 8 | 10 | 12 | . . . |
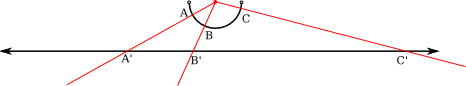
It is also possible to compare some infinite sets geometrically -- using geometry to show how points in the sets can be paired up. A geometric argument can be used to show that the points on a short semicircle (not including the endpoints) can be paired up with the points on an infinitely long line:

To set up the pairing as shown here, take any point, X, on the semicircle. Draw a ray from the center of the semicircle through the point X on the semicircle. This ray will eventually intersect the infinite line, in a point X'. We simply pair up X with X'. In the picture, three such pairs are shown: A/A', B/B', and C/C'. Since we can pair up any point on the semicircle with a point on the infinite line, with none left over in either set of points, we see that the two infinite sets of points have the same size.
Remarkably, once we accept this way of comparing the sizes of infinite sets, it turns out that not all infinite sets have the same size. This fact was discovered by Georg Cantor in the nineteenth century. In fact, Cantor showed that there are infinitely many different sizes of infinity. This is a strange result, and Cantor's theory of infinity was very controversial at first.
As an example, we can compare the set that contains the positive integers to the set that contains all the subsets of positive integers. The items in the latter set are themselves sets, such as { 17 }, { 1, 2, 3, 4 }, { 2, 4, 6, 8, ... }, and the set of prime numbers. One can show, as we did in class, that it is impossible to pair up the items in the set of positive integers with the items in the set of subsets. This shows that the set of subsets of positive integers is not the same size as the set of positive integers. The set of subsets is in fact a strictly bigger infinity
Cantor was also able to show that the set of all decimal numbers (such as 3.17, 42.0, 1.33333..., π, and the square root of two) is is strictly bigger infinity than the set of positive integers. This is probably his most famous result.
Exercise 1: Show, with a diagram, that the set of positive integers { 1, 2, 3, 4, 5, ... } can be paired up with the set { 2, 3, 4, 5, ... }, which contains all the integers greater than 1. Then consider the following definition of "infinite": A set is infinite if removing an item from the set does not change the size of the set. Explain in words why this is a reasonable definition. (What happens with finite sets?)
Exercise 2: Show, with a diagram, that the set of positive integers { 1, 2, 3, 4, 5, ... } can be paired up with the set { ..., -2, -1, 0, 1, 2, 3, ... }, which contains all the integers, positive, negative and zero. (You will have to rearrange the items in the latter set.)
Exercise 3: Show geometrically (by drawing a picture and explaining what you have done) that the number of points on a one-inch line segment is the same as the number of points on a two-inch line segment.
Exercise 4: Show geometrically (by drawing a picture and explaining what you have done) that the number of points on a big circle is the same as the number of points on a small circle.
Exercise 5: "Draw a line segment, and take a look at it. It contains an infinite number of points, right? And it's an actual infinity, right there before your eyes, right? So why is there any question about whether actual infinities exist?" Write a short essay to discuss what is right and/or wrong about this argument. (Hint: Consider the atomists.)