
This week's assignment is based on Section 4.2 of Symmetry, Shape, and Space and on the handout on number systems that was given out in class. This is an individual assignment. You can work on the assignment with other people in the class, but you should write up your own responses to the exercises to turn in. The assignment is due in class on Wednesday, October 1.
Reminder: There is a test on Friday, October 3
Part 1. Write each of the following numbers using the Egyptian system, the Greek system, and the Babylonian system that were discussed in the handout. (Don't just give an answer; show how the answer is obtained.)
(a) 5 (b) 42 (c) 137 (d) 2008 (e) 72331
(Hint: 2008 = 33*60 + 28, and 72331 = 20*3600 + 5*60 + 31.)
Part 2. Translate each of the following numbers into our own number system. (Don't just give an answer; show how the answer is obtained.)
(a) ιδ ω ζ
(b) 
(c) 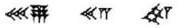
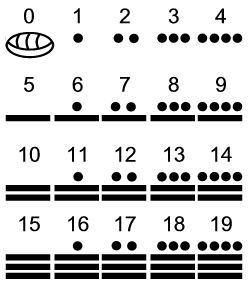
Part 3. The Maya, in pre-Columbian Central America, used a number system that was similar to the Babylonian, except that it used 20 as its number base instead of 60. A picture of the twenty basic numerals in the Mayan system is shown below. (They has a rather surprising alternative set of numbers that you will encounter later in the course.) Write a short essay that explains both how the 20 basic numerals are constructed and how the Mayan system would be used to represent larger numbers. (What do the "dot" and "bar" represent?) Include some examples of expressing numbers in the Mayan system.
Part 4. Section 4.2 in Symmetry, Shape, and Space includes a discussion of "reptiles." A rep-tile is a shape such that several copies of the shape can be put together to make a larger version of the shape. Exercises 4 and 6 in Section 4.2 deal with two examples of reptiles. Do Exercises 4 and 6 from Section 4.2.
Part 5. Section 4.2 covers several techniques for making irregular shapes that tile the plane. One of the techniques is to start with a simple tile that has parallel sides, such as a parallelogram or hexagon, and then to apply parallel translation to one of more pairs of parallel sides. Exercises 9 and 10 in that section ask you to make irregular tilings by applying parallel translation to all pairs of parallel sides in a parallelogram or hexagon. Do one of Exercise 9 and Exercise 10 from Section 4.2. You can draw your tiling either on tracing paper or normal paper. Grading will be based partially on artistic merit.
Part 6. Section 4.2 covers three additional methods for producing irregular tiles: glide reflection, midpoint rotation, and side rotation. Exercises 12, 13, and 14 ask you to create tilings that use various combinations of these methods. Do any one of Exercises 12, 13, and 14 from Section 4.2. You can draw your tiling either on tracing paper or normal paper. Grading will be based partially on artistic merit.